WOW!!!
- Thread starter fatman
- Start date
- Status
My wife showed me one of those videos where they did it from the camera looking down. To see the changes in the wave is awesome.
That is an excellent example of "harmonic progression" and pendulum theory.
Very cool.
Thanks for the link.
Very cool.
Thanks for the link.
I am dealing with something very similar to this in my calc based physics class right now. It looks cool but is a pain in the ass to deal with on paper! Trust me on that.
I'm in Calc 3 and Physic 2 right now. I have to say that it is very interesting, but I will be happy to get it done. Oh, I am taking a Java class on top of all that, so I'm pretty tired.
@ Jason..... Let me bore you for a minute or two. (hazards of a conservatory styled music education)
It has to do with the "amplitude". While the distance traveled is the same, the frequency (period) is determined by the length of the pendulum. If the weight is consistent, the shorter the pendulum the faster it will travel. This is consistent with the harmonic progression of sound. The patterns produced by the differing lengths of the pendulums are predictable and repeatable. W1nd0wl1cker stated that the patterns resemble something similar to what he is studying. (This maybe?)
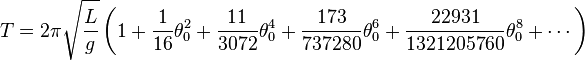
The harmonic progression is consistent (the patterns), and inherently similar to the properties of the pendulum. If one pendulum is twice the length of a second and the amplitude is the same then the second will swing twice as fast and the first. If several are set in motion, and each is a specific length based on the shortest and the longest, and all have the same amplitude a consistently changing pattern will be produced. Which will after a full cycle of oscillations repeat the series of patterns again. But, the effects of gravity will diminish the amplitude (over time) that will result in all pendulums coming to rest. The patterns produced will be consistent until rest is achieved.
The phenomenon is similar to what happens when two tones are sounded at the same time. The oscillations of each tone will become synchronous and un-synchronous, and will produce an upper and lower tones based on the harmonic series. When the tones are synchronous the volume is increase and will be perceptively louder... it is the additive quality of sound... But, pendulums are not additive and will not increase in amplitude, only diminish.
Note: at the half way point (give or take) the pattern is split into two rows of pendulums... from that point on the pattern reverses itself and progresses back to the original line, then begins again. But, is still considered to be progressive.
Pendulum
It has to do with the "amplitude". While the distance traveled is the same, the frequency (period) is determined by the length of the pendulum. If the weight is consistent, the shorter the pendulum the faster it will travel. This is consistent with the harmonic progression of sound. The patterns produced by the differing lengths of the pendulums are predictable and repeatable. W1nd0wl1cker stated that the patterns resemble something similar to what he is studying. (This maybe?)
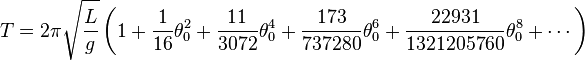
The harmonic progression is consistent (the patterns), and inherently similar to the properties of the pendulum. If one pendulum is twice the length of a second and the amplitude is the same then the second will swing twice as fast and the first. If several are set in motion, and each is a specific length based on the shortest and the longest, and all have the same amplitude a consistently changing pattern will be produced. Which will after a full cycle of oscillations repeat the series of patterns again. But, the effects of gravity will diminish the amplitude (over time) that will result in all pendulums coming to rest. The patterns produced will be consistent until rest is achieved.
The phenomenon is similar to what happens when two tones are sounded at the same time. The oscillations of each tone will become synchronous and un-synchronous, and will produce an upper and lower tones based on the harmonic series. When the tones are synchronous the volume is increase and will be perceptively louder... it is the additive quality of sound... But, pendulums are not additive and will not increase in amplitude, only diminish.
Note: at the half way point (give or take) the pattern is split into two rows of pendulums... from that point on the pattern reverses itself and progresses back to the original line, then begins again. But, is still considered to be progressive.
Pendulum
One other small point... You may have noticed that the line of weight/bobs were not in a straight line, but was slightly curved. If the line of bobs was straight it would be analogous to the diatonic system of tones we use in our "Western" style of music. Since the natural harmonic progression isn't diatonic our music is actually out of tune. Eastern music (traditional Japanese for example) and microtonal music is based on the natural harmonic progression.
Just incase you were wondering...
I know.... he's :crazy:
Just incase you were wondering...
I know.... he's :crazy:
Thanks Lawrence, for saving me the hassle of having to explain all that.
Although I have no clue what you actually said, that was fascinating.
Although I have no clue what you actually said, that was fascinating.
- Status